Modulation Transfer Function (MTF) and Subjective Quality Factor (SQF)

Part 4: Subjective Quality Factor

SQF was developed by Grainger et al in the 1970s [1] to provide a more easily understandable measure of image quality which takes into account not only the intrinsic quality of a lens, but also the nature of how we see things.

It has been demonstrated by experiment that human vision has a bandpass characteristic whereby we see some spatial frequencies better than others. Just like a lens, the higher the spatial frequency of the detail we are looking at, the more difficult it is to resolve. However unlike a lens, the human visual system also doesn't see very low spatial frequencies well. The high frequency falloff is probably due to the optical limitations of the eye's lens, but the low frequency falloff is due to the physiology of the retina and the way the brain interprets visual information. So the human visual system can be considered to have an "MTF" which peaks as shown below:

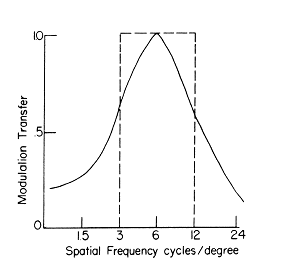
As you can see there is a peak at 6 cycles per degree. This translates to 1 cycle per mm for a print that is viewed at a distance of 34cm (about 13.5"). What this means is that when you view a print from that distance, which would be typical for close viewing, the MTF at around 1 cycle/mm in the print would have the most influence on whether you thought the print was sharp or not. Grainger found that he could correlate the subjective impression of sharpness with the MTF of the spatial frequencies in the print that correspond to the human visual response between 3 and 12 cycles per degree which turns out to be from 0.5 to 2 cycles per mm in the print when viewed at a distance of 34cm.

Grainger then developed a model, which basically states that the subjective sharpness of a print corresponds to the area under the MTF curve between the spatial frequencies of (0.5 x magnification) and (2 x magnification) when spatial frequency is plotted on a logarithmic scale. He verified this model by making prints using optical system with known MTFs and asking viewers to rate the images in terms of sharpness and he found very good agreement between his calculated SQF values and the subjective rating of image quality by viewers.

So to give a concrete example, if we make an 8x10 (or 8x12) print from a 35mm negative we have to magnify the negative by a factor of 8 (since the negative is approximately 1" x 1.5"). So as far as the SQF is concerned, the area under the MTF curve of the lens between 4 and 16 cycles/mm is then what really counts. The higher the MTF is in that region, the higher the print quality will appear to be and the higher the SQF will be.

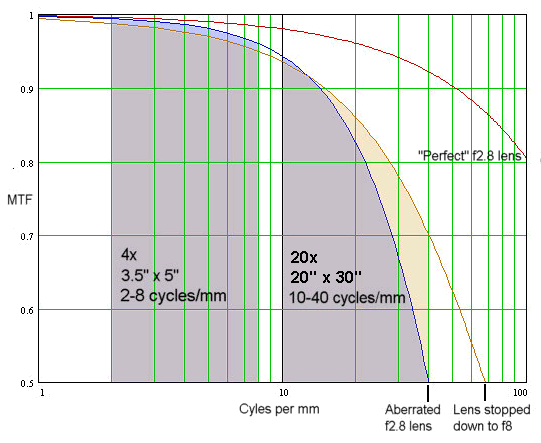
This figure shows how MTF and SQF are related. The MTF is plotted for a "perfect" f2.8 lens (diffraction limited, no aberrations), a real f2.8 lens operating wide open (blue trace) and that same lens stopped down to f8 (brown trace). At f8 this lens isn't quite perfect (it's not diffraction limited), but residual aberrations are very small. For a 4x enlargement to a 3.5 x 5 print the SQF is related to the area under the MTF curve as shown on the left. As you can see, the area under the blue curve is very slightly greater than that under the brown curve, though lenses would probably produce an equally good print. That's reflected in most lens tests where almost all lenses get an A+ rating for 3.5 x 5 prints.

The area on the right represents a 20x enlargement to a 20 x 30 print. This time you can see two things. First the shaded area is smaller than that for the 4x print, resulting in a lower SQF. A 20x print won't look as good as a 4x print. Second you can see that now the lens at f8 has a slight advantage. Prints made with the lens stopped down to f8 will appear sharper.

This data also shows something you might not expect, that, on the basis of SQF, it's possible for lens "A" to be better than lens "B" for small prints, but lens "B" may be better than lens "A" for large prints. Normally this doesn't happen, but it is possible. It also follows from this that it's possible that prints from lens "A" may look better than prints from lens "B" when viewed from a distance, but when viewed closely prints from lens "B" may look better than prints from lens "A"! This would be an unusual situation, but it could happen.

Interestingly, in the 1980s a Swedish research group showed that subjective image quality could even be predicted from a simpler method that looked only at the MTF at a single spatial frequency rather than over a range of spatial frequencies as Grainger's model does [2]. However Grainger's model probably applies to a wider range of lenses with a wider range of aberrations and so may give a more accurate prediction.

[NEXT: SQF and Popular Photography]