

Bokeh and Background Blur
While sharpness is an essential and sought after part most photographs, the creative use of background blur (and Bokeh) can often be a very useful compositional element.
There are two main properties of background blur, the shape (or quality) and the size (or quantity).
Blur Quality - Bokeh
The quality of the blur has come to be known as "bokeh". The origin of the
term is from the Japanese word ![]() which in romaji (english characters) is spelled
"boke" ( pronounced bo-keh) and which means "fuzzy", but usually in
the sense of "touched in the head" or "senile". It can also be used to
describe someone who says stupid things, or makes silly mistakes. However in the world of
photography Bokeh has come to be used to refers to the fuzzy or confused nature of out of
focus areas in photographs. Are they smooth and uniform or are they some other shape and
texture? Smooth, uniform, aesthetically pleasing blur is "good" Bokeh
while blur which shows evidence of ugly shape and structure is "bad" Bokeh.
which in romaji (english characters) is spelled
"boke" ( pronounced bo-keh) and which means "fuzzy", but usually in
the sense of "touched in the head" or "senile". It can also be used to
describe someone who says stupid things, or makes silly mistakes. However in the world of
photography Bokeh has come to be used to refers to the fuzzy or confused nature of out of
focus areas in photographs. Are they smooth and uniform or are they some other shape and
texture? Smooth, uniform, aesthetically pleasing blur is "good" Bokeh
while blur which shows evidence of ugly shape and structure is "bad" Bokeh.
For good Bokeh, ideally points and lines would blur smoothly as they fell out of focus, in the manner, for example, of a smooth Gaussian blurring. This is illustrated by the left and right images below. The left image shows a pattern which is in focus and the right image shows what an ideal gaussian blurring might look like.
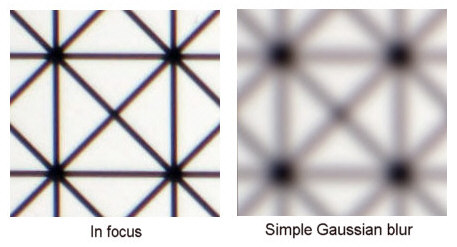
However, that's not the way optics work. Blurring does not occur in a smooth Gaussian manner as objects fall out of focus. What happens is shown below:
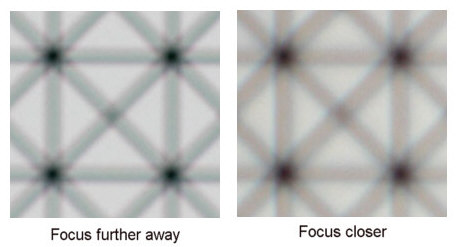
These are examples what you get when the focus isn't on the pattern, but is adjusted to be either further away (left) or closer (right). The lines don't blur smoothly and can interact to give patterns as is shown by the star shaped and square structures that appear where the lines cross. Aesthetically these patterns are less pleasing than a smooth Gaussian blur would be and so would not be classified as examples of good bokeh. In this case chromatic effects are also visible and the color of the blur patterns is different inside and outside focus.
The exact nature of the out of focus pattern depends on a number of things, but mainly on the design of the lens and the manner in which aberrations are controlled in the out of focus image. While all lenses designs attempt to minimize aberrations in the in focus image, different designs will have different levels and types of aberration in the out of focus image and thus yield images with different bokeh.
Below are examples (calculated) showing the structure of the image of an infinitely small point (such as the image of a star) under various focus conditions, with and without aberrations. These are what you would see at very high magnifcation of the image (at much higher resolution than any film or digital camera can record). A real image can be though of as being made up of a very large number of such spots. As you can see, even at the microscopic level the blurring is not smooth. The structure in these points is due to the wave nature of light and interference phenomena.

The shape of the aperture is also a factor in determining bokeh. The further from circular the aperture is, the more the out of focus image is likely to show poor bokeh. The shape of the aperture is also reflected in the shape of out of focus images of small areas of light. This is shown quite dramatically below in a series of images of an out of focus highlight in an image.

On the left is the defocused image in the center of the frame taken with a 50mm f1.8 lens, wide open at f1.8. In this case the out of focus image is circular. However notice also that it's not a smooth blurring of a point source as you'd like for ideal bokeh It's pretty uniform in illumination and actually appears to have a brighter ring around the outside. In the middle is the defocused image also at f1.8 , but in the corner of the frame. This time the out of focus image is lenticular in shape. This is due to how the aperture looks to oblique light rays and the shape is caused by the same factors that result in vignetting in the corners of an image when a lens is used wide open.
This particular lens has 5 aperture blades, so when it is stopped down the aperture is pentagonal. In the image on the right this can quite clearly be seen in the out of focus image. Again this is likely lead to less pleasing bokeh than a circular aperture would. Better lenses often have more aperture blades, and those blades are often curved so as to give an aperture that's closer to being circular. Very cheap cameras may use 4 or even 3 blade apertures resulting in square or triangular out of focus highlight which do not create good bokeh.
Note though that a perfectly round aperture is no guarantee of good bokeh. All lenses used wide open show a circular out of focus points in the center of the image, though not all lenses have good bokeh. The out of focus aberrations also have a significant effect.
The classic example of aperture shape yielding unappealing bokeh is the mirror lens. The aperture of a mirror lens is donut shaped, a circular aperture with a circular blockage right in the center as shown below in a defocused star image

This "donut" shape can often clearly be seen in defocused areas of images shot with mirror lenses, as shown in the following pair of images. The image on the left was shot using a 500mm f8 mirror lens, while the image on the right was shot using a conventional refractive 500mm lens at f8. I think most people would agree that the background blur in the image shot with the mirror lens is less attractive than that shot with the conventional refractive lens.

Photographers will often argue about the quality of the bokeh for a particular lens. This is probably because the out of focus image quality depends a lot on exactly how far out of focus the image is, and whether the blur results from objects being closer or further away than the plane in focus. The same lens may show different bokeh when used in different situations with foreground and background objects at different distances. So when comparing two lenses it's quite possible that one will produce more pleasing bokeh under one set of conditions, but the other lens may be better under a different set of conditions. Some confusion also comes from the fact that differences in bokeh can be pretty subtle at times. Below is a comparison of two 50mm lenses at f2.0 and f2.8. These images are crops from the full size images show the same region of out-of-focus foliage. I think most people would say that lens "B" has the better bokeh. It's slightly smoother, especially at f2.0, but unless they were looking closely, such differences probably wouldn't be obvious to most people when looking at a print.

For the terminally curious, in this case lens "A" was a Canon EF 50/1.8 and lens "B" was a Pentax 50/1.4 in an M42 screw mount, mounted on the EOS body via an adapter
Blur Quantity
While the quality of blur - bokeh - is in itself a "fuzzy" concept and something that's quite difficult to predict or control, the quantity of blur can be calculated quite easily and it's something over which the photographer has control through choice of focal length and aperture.
Most photographers are familiar with the concept of depth of field. It's the range of distances over which objects are rendered acceptably sharp. It might be natural to assume that the smaller the depth of field, the more blurred objects outside the depth of field would be, but that would be an incorrect assumption.
It is true that the amount of blurring of objects that are close to but just outside the region in focus is greater when the depth of field is smaller, but it's not true for distant objects. What determines how blurred distant background objects are is the physical size of the lens aperture. This is simply the focal length divided by the f-stop, so, for example, a 50mm lens used at f4 has a physical aperture of 50/4 = 12.5mm.
For a lens that's focused on a nearby subject and when the depth of field is fairly small:
- The degree of blur of objects close to the main subject is determined by the f-stop of the lens in use. The faster the lens, the smaller the depth of field, the greater the local blur.
- The degree of blur of objects FAR behind the subject is given by the physical size of the aperture of the lens in use.
- The degree of blur at intermediate distances behind the subject has to be calculated. There are no simple rules, except that it's more for fast lenses with large physical apertures.
This is probably best shown by an example. Let's say you're taking a portrait and you wonder whether a 50/1.4, 85/1.8, 135/2 or 135/2.8 will better isolate the subject by blurring the background. To keep thing fair, longer focal length lenses are used from further away to keep the subject size (magnification) the same in each case. The size of the blur is the size that the defocused image of a point would be on the camera sensor. A DSLR with a 1.6x multiplier APS-C sensor is assumed (e.g. Canon EOS 30D) for depth of field calculations.
| 50mm @ f1.4 | 85mm @ f1.8 | 135mm @ f2 | 135 @ f2.8 | |
| Distance to subject | 2.05m | 3.5m | 5.5m | 5.5m |
| Field of view | 24" x 36" | 24" x 36" | 24" x 36" | 24" x 36" |
| Magnification | 0.025x | 0.025x | 0.025x | 0.025x |
| Physical Aperture | 35.7mm | 47.2mm | 67.5mm | 48.2mm |
| Depth of field | 9.73cm | 11.2cm | 12.5cm | 17.5cm |
| Blur 25cm behind subject | 0.097mm | 0.079mm | 0.073mm | 0.052mm |
| Blur 50cm behind subject | 0.18mm | 0.15mm | 0.14mm | 0.1mm |
| Blur 1m behind subject | 0.29mm | 0.26mm | 0.26mm | 0.18mm |
| Blur 3m behind subject | 0.53mm | 0.56mm | 0.59mm | 0.42mm |
| Blur 10m behind subject | 0.74mm | 0.87mm | 1.1mm | 0.78mm |
| Blur at Infinity | 0.89mm | 1.18mm | 1.69mm | 1.21mm |
As you would expect, at the same magnification, the faster the lens, the smaller the depth of field. This also means that the background close to subject will be blurred more by the faster lens. In this case the 50mm lens at f1.4 gives slightly greater blurring for objects up to about 1m behind the subject in focus. However as you go further back, the lens with the largest physical aperture starts to show the most blur, and by the time you're at infinity, the 135mm lens at f2 lens will give almost twice as much blurring (actually 1.9x as much). The following images show the effect quite clearly. All three were shot to produce the same magnification of the camera box and so have essentially the same depth of field (region of "acceptably sharp" focus), but the image shot with the larger physical aperture (longer focal length) lens shows the greatest degree of background blur.

Calculating the magnitude blur is a little tricky but I've written a simple program which will do the calculation for you. It calculates both foreground and background blue (as well as depth of field and magnification) and it can be downloaded from an earlier page I wrote on this subject: Background Blur Calculator
